.
A typical integer 
has 
digits, half of
which one expects to be 1's, so that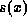 
should be
approximately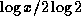 
. Therefore, we compare 
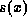with
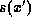, when 
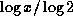and 
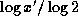have the same
fractional part, by considering the function
In 1968, Trollope proved the following result:
Let .
A typical integer has digits, half of
which one expects to be 1's, so that 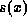should be
approximately 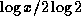. Therefore, we compare 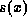with
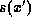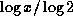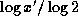, when and have the same
fractional part, by considering the function
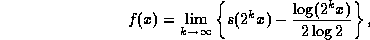
for each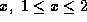 
.
One can easily show that this limit exists and
that the function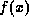 
is continuous. However Trollope
proved the surprising result that 
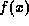is nowhere differentiable.
For more on such questions see the paper by Boyd, Cook and Morton.