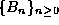, and the Bernoulli
polynomials, 
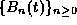, are defined by the power series
The Bernoulli numbers, 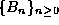, and the Bernoulli
polynomials, 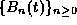, are defined by the power series
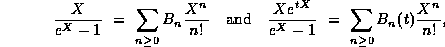
so that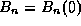 
and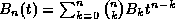 
.
Some useful facts, that follow straight from these definitions, are that
each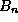 
is a rational number, 
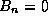if n is odd 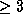and 
, and
(28)
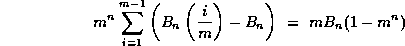
for all integers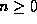 
.
In 1840 Clausen and Von Staudt showed that the denominator of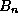 
(n even)
is precisely the product of those primes p for which p-1 divides n;
and further that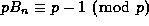 
for each such p
(actually one also has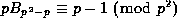 
).
In 1851 Kummer showed that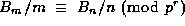 
for any even integers m and n, satisfying
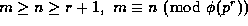and
; and one can use this in showing that
(29)
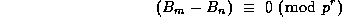
whenever 
and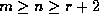 
.
For any positive integers t and n, we have
(30)
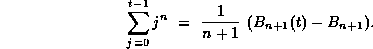
and Kummer's congruences for Bernoulli polynomials.
Consequently, if
m divides up+v, for given integers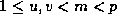 
, then,
by combining the identity
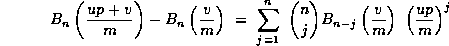
with (30), we obtain
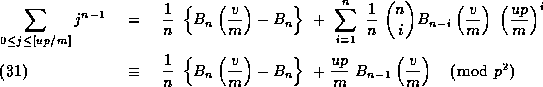
for primes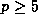 
provided 
.